|
It is important to remember that the natural logarithm function, ln(x),
and the natural exponential function, ex, are inverse functions.
When a function is composed with its inverse, the starting value is returned.
ln(ex) = x and eln(x) = x
When studying ex, some people find it easier to express ex, as exp(x),
so that the composition of functions is more
clearly observed.
ln(exp(x)) = x and exp(ln(x)) = x
Examples:
| |
Simplify: |
Answer |
1. |
ln(ex) |
Knowing that ln(x) and ex are inverse functions, the simplification under composition is x.
ln(ex) = ln(exp(x)) = x |
2. |
ln(e) |
Noting that the exponent on e is 1 (the x-value is 1), and applying what we just saw in #1, we know the simplification is one.
ln(e) = ln(exp(1)) = 1 |
3. |
eln(x) |
Again, we know that ln(x) and ex are inverse functions, so the simplification under composition is x.
eln(x) = exp(ln(x)) = x |
4. |
eln(7) |
Noting that the x-value is 7,exponent on e is 1, and applying what we just saw in #3, we know the simplification is seven.
eln(7) = exp(ln(7)) = 7 |
5. |
e3ln(4) |
 That "3" is interfering with the composition of the inverse functions. Move the "3" by using the log property that
ln ar = r ln a.
|
|
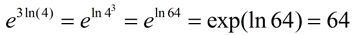
For help with exponential expressions on your calculator, click here.
|
|
NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|
|
|
