|
Directions: Read carefully. You should be able to solve these questions without your graphing calculator. Choose the best answers.
1. |
Given: f (x) = (2x + 1)(x + 3)(x - 2)
For what values of x is f (x) > 0 ?
(Check all that apply, and hit SUBMIT!) |
 |
|
2. |
Regarding the graph at the right, the function is: |
|
|
| |
|
3. |
Given: f (x) = (2x + 1)(x + 3)(x - 2)
How many relative maxima does this function have?
|
|
|
Choose:
|
|
4. |
A fifth degree polynomial function is shown below.
Which of the statements are NOT true about this function?
(Check all that apply, and hit SUBMIT!) |
|
|
5. |
Given h(x) = -(x - 1)(x + 2)(x - 5)
What are the end behaviors of the graph of this function?
Choose:
|
| |
|
6.
|
Which of the following polynomial functions could be represented by the graph at the right?
Choose:
|
|
|
7. |
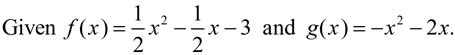
Which of the statements are true about these functions?
(Check all that apply, and hit SUBMIT!) |
|
|
8. |
Which of the following functions represents a polynomial function with degree 3, roots x = 0, x = -1 and x = 2, and with end behavior approaching positive infinity as x approaches negative infinity?
Choose:
|
|
9. |
Given: f ( x) = ( x + 1) 2(3 - x)
Which of the following statements is FALSE regarding its graph?
Choose:
|
|
|
10. |
Given: f (x) = x2 - 16
On which interval(s) is this function positive?
Choose:
|
|
|

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|