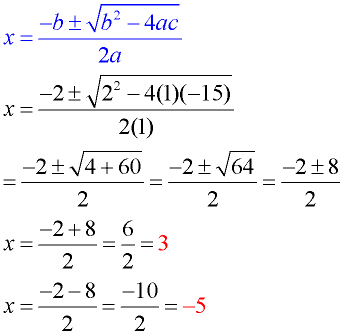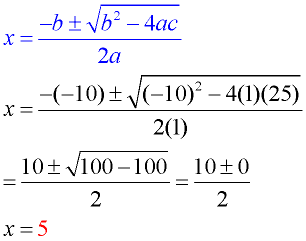refresher
Look out! Our math ninjas are back!!

quadguy
quad button
quadformguy2

The solutions for some quadratic equations are not rational, and cannot be obtained by factoring.
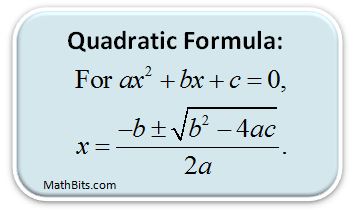
The quadratic formula, however, may be used to solve ANY quadratic equation (even the ones that can be factored). This is a formula that you want to know and remember!

reminder
• As you can see in the formula, the coefficients (numbers) "a", "b", and "c" from
    ax2 + bx + c = 0 are substituted into the formula.
• Also notice that the formula will yield two (±) solutions, since a quadratic is a
    second degree equation.
• The "2a" in the denominator is underneath the entire top, not just the radical.
• Be careful with the "signs" of the "a", "b", and "c" values in your calculations.

Let's see the Quadratic Formula at work in various situations:

expin1    Solve: x2 + 2x - 15 = 0
Also factorable.
Solution using Quadratic Formula: a = 1; b = 2; c = -15
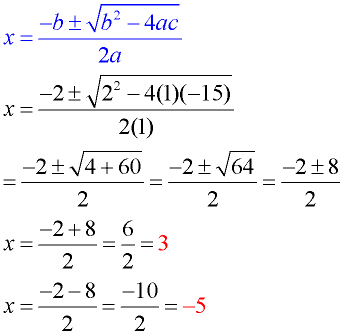
quadform1
This equation is also factorable.
(x + 5)(x - 3) = 0
x = -5; x = 3

Notice that the quadratic formula ALSO gives the correct results.

beware1



expin1   Solve: 2x2 - 10x = -3
Not set equal to zero!
Set equation equal to zero: 2x2 -10x + 3 = 0
Solution using Quadratic Formula: a = 2; b = -10; c = 3
quadform2a
quadform22b
Not factorable.

Notice the needed parentheses for dealing with the "b" value of "-10".

quadbeware2a
Radical answers are"exact" answers.
Decimal answers are
"approximate" answers.



expin1   Solve: x2 - 6x + 13 = 0
Negative under radical!
Solution using Quadratic Formula: a = 1; b = -6; c = 13

quadform3
Not factorable.

quadbeware2

quadform3a



expin1   Solve: x2 -10x + 25 = 0
Repeated answer!
Solution using Quadratic Formula: a = 1; b = -10; c = 25
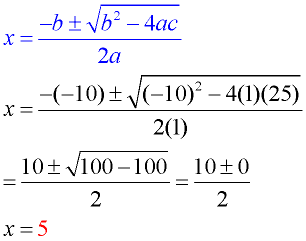
quadform4
Be careful here! It appears that there is only ONE answer, but this is actually a "repeated" root. The graph is tangent to the x-axis at x = 5.
This equation is also factorable.
(x - 5)(x - 5) = 0
x = 5; x = 5
Answer repeats.
beware4

 

divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".