A radical expression is one which contains a root (square root, cube root, etc.).
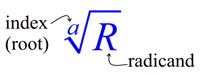 
When first working with radicals, the expressions are primarily limited to
square root (a = 2) expressions and cube root (a = 3) expressions.
 Let's examine Square Roots:
[On this page, the radicand will be non-negative. No negatives under the radical.]
Square roots have an index value of two. When you see a radical with no index listed, it is assumed to be an index of two, a square root. Let's examine Square Roots:
[On this page, the radicand will be non-negative. No negatives under the radical.]
Square roots have an index value of two. When you see a radical with no index listed, it is assumed to be an index of two, a square root.
|
A square root is in simplest form when
1.
the radicand contains no perfect square factors
2. the radicand is not a fraction
3. there are no radicals in the denominator of a fraction. |
 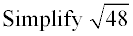
1. Find the largest perfect square
factor (the largest perfect square that divides into 48 with no remainder). You need to be familiar with a list of perfect squares.

2. Give each factor its own radical sign. 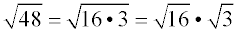
3. Reduce the "perfect square" radical that was created. 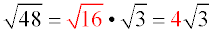
4. ANSWER: 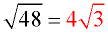
Don't worry if you do not pick the LARGEST perfect square factor to start. You can still get the correct answer, but you will have to repeat the process. See what happens if we choose 4 instead of 16 to start:
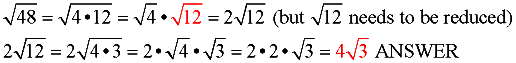
Notice how the out-front 2 in the second line is multiplied along for the rest of the problem.
|
 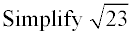
The number 23 cannot be factored by any of the perfect squares (23 is prime). This is a trick question as it is already in simplest form and cannot be reduced further.
This process of removing a radical from the denominator is referred to as "rationalizing the denominator" because it turns the denominator into a rational (not irrational) value.
 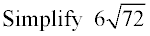


The square root of a value is a quantity which, when squared, equals the radicand (the number under the square root symbol.) For example, the square root of 16 could be either + 4 or -4, since both, when squared, equal 16.
It is understood, however, that the square root (radical) symbol denotes only the positive root, called the "principal square root". 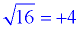 When solving the equation x2 = 25, you are searching for both solutions: +5 and -5. So, we write: 
|
 Let's see how this approach works with Cube Roots: Let's see how this approach works with Cube Roots:
 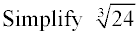
1. Find the largest perfect cube
factor (the largest perfect cube that divides into 24 with no remainder).
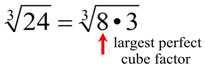
2. Give each factor its own radical sign. 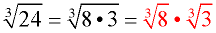
3. Reduce the "perfect cube" radical that was created. 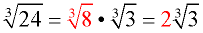
4. ANSWER: 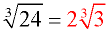
|
Perfect Squares
4 = 2 x 2
9 = 3 x 3
16 = 4 x 4
25 = 5 x 5
36 = 6 x 6
49 = 7 x 7
64 = 8 x 8
81 = 9 x 9
100 = 10 x 10
121 = 11 x 11
144 = 12 x 12
169 = 13 x 13
196 = 14 x 14
225 = 15 x 15 |
Prime Factorization
Radicals can also be simplified by expressing the radicand using prime factorization and looking for groups of two similar factors to form a perfect square factor.
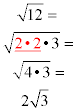
|
Product Rule
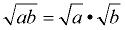 where a ≥ 0, b≥ 0
where a ≥ 0, b≥ 0
"The square root of a product is equal to the product of the square roots of each factor."
This theorem allows us to use our method of simplifying radicals.
|
Quotient Rule
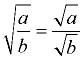
where a ≥ 0, b > 0
"The square root of a quotient is equal to the quotient of the square roots of the numerator and denominator."
|
Perfect Cubes
8 = 2 x 2 x 2
27 = 3 x 3 x 3
64 = 4 x 4 x 4
125 = 5 x 5 x 5
|
Remember:
When working with radicals, the term "simplify" means to find an equivalent expression.
It does not mean to find a decimal approximation. |
|