In this section, we will concentrate on examining "algebraic" square roots.
Let's see what happens when algebraic variables are involved.
 Algebraic Square Roots:
[On this page, all variables represent positive values, and the radicands are non-negative).] Algebraic Square Roots:
[On this page, all variables represent positive values, and the radicands are non-negative).]
 Square Roots
Square Roots |
Radicals that are simplified have:
1. no fractions left under the radical.
2. no perfect power factors under the radical.
3. no exponents under the radical greater than the index value.
4. no radicals appearing in the denominator of a fractional answer.
|
Before we begin, take a minute to look at the first table at the right called "Perfect Squares". Notice how variables are perfect squares when their exponents are even numbers.
Also, remember the exponent rules, xa • xb= xa + b and (xa)b = xab.
 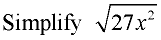
1. First, we will separate the number value from the algebraic variable. This will give us a chance to examine each for perfect square factors.

2. Give each factor its own radical sign. 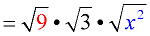
3. Reduce the "perfect square" radicals. 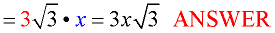
 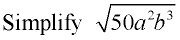
Separate and find the largest perfect square factors.
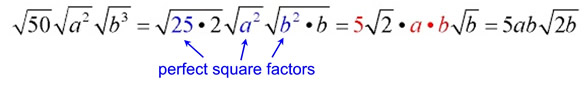
 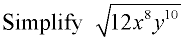
Separate and find the largest perfect square factors. Remember that even numbered exponents are perfect squares.

 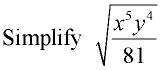

The quotient rule was applied and the perfect square factors found.
|
Perfect Squares
x2 = x•x
x4 = x2•x2
x6 = x3•x3
x8 = x4•x4
Powers are even.
|
Product Rule
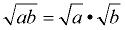 where a ≥ 0, b≥ 0 where a ≥ 0, b≥ 0
"The square root of a product is equal to the product of the square roots of each factor."
This theorem allows us to use our method of simplifying radicals.
|
Quotient Rule
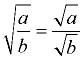
where a ≥ 0, b > 0
"The square root of a quotient is equal to the quotient of the square roots of the numerator and denominator."
|
Perfect Squares
4 = 2 x 2
9 = 3 x 3
16 = 4 x 4
25 = 5 x 5
36 = 6 x 6
49 = 7 x 7
64 = 8 x 8
81 = 9 x 9
100 = 10 x 10
121 = 11 x 11
144 = 12 x 12
169 = 13 x 13
196 = 14 x 14
225 = 15 x 15
|
|