|
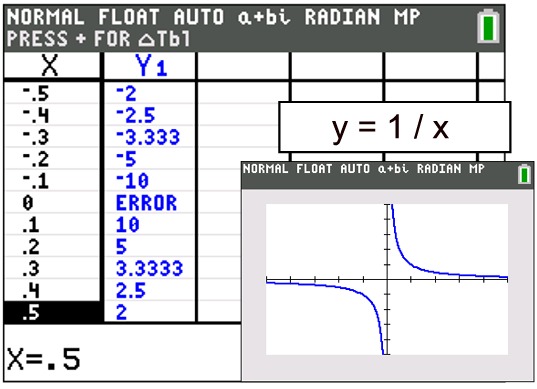
Let's start by examining the graph of a simple rational function. You may have seen this graph in the past. Due to the restrictions that are placed on the x-values of rational functions, you can guess that their graphs are going to be more complicated to predict around these undefined locations.
 ; x ≠ 0 ; x ≠ 0
Notice, in the table, the behavior of the graph around its undefined value at x = 0.
The function changes its sign. Its values are negative to the left of 0, but they are positive to the right of 0. |
|
|
|
Also, notice what is happening to the graph surrounding x = 0.
• As the graph approaches x = 0 from the right, its y-values are getting increasingly larger.
As
x → 0+, y → + ∞.
( 0+ approaches from right, 0- approaches from left)
• As the graph approaches x = 0 from the left, its y-values are getting increasingly smaller.
As x → 0-, y → - ∞.
• x = 0 is a vertical asymptote for this graph. |
|
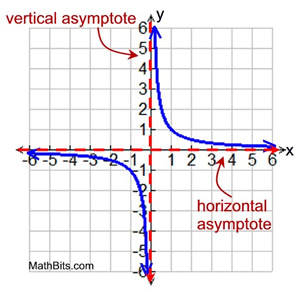
A vertical asymptote is a vertical straight line toward which a function approaches closer and closer, but never reaches (or touches). |
Vertical asymptotes correspond to the undefined locations of rational functions. |
Also, notice how the graph is "approaching" the x-axes at the far right and far left.
• As x gets increasingly larger, (x → ∞), the y-values are positive and are getting closer and closer to 0 (the x-axis), but they will never actually get to zero. They will just continue to get closer and closer to zero (the x-axis).
• As x gets increasingly smaller, (x → - ∞), the y-values are negative and are also getting closer and closer to 0 (the x-axis), but they will never actually get to zero. They will also just continue to get closer and closer to zero.
• y = 0 is a horizontal asymptote for this graph.
The behavior of the graph in relation to a vertical asymptote is clear cut and constant: the graph NEVER touches them. Never!
The behavior of the graph in relation to a horizontal asymptote, however, is not as clearly defined. You may only approach a horizontal asymptote, or you may touch a horizontal asymptote, or you may even cross a horizontal asymptote. Horizontal asymptotes give more of a general impression of what the graph is doing, and are generally associated with the far ends of the graph. |
|
Don't Panic!!!
In Algebra 2, you only need to have a general understanding of this type of graph so you will know what you are seeing on your graphing calculator, and how the graph relates to the restrictions on a rational function.
|

How to sketch a rational function without a graphing calculator:
(and yes, this will be a "rough", but sufficient, sketch)
| 1. As with all functions, find the intercepts: |
| |
• for the x-intercept set y = 0
• for the
y-intercept set x = 0 |
Beware: As we saw in the graph above,
rational functions may not have any intercepts. |
| 2. Find the vertical asymptote(s): |
| |
• set the denominator = 0 and solve |
3. Find the horizontal asymptote(s): (assuming the rational function is expressed as a single fraction)
|
| |
• get the degree of the numerator, n, such as axn
• get the degree of the denominator, m, such as bxm
• if n < m, the x-axis is the horizontal asymptote
• if n = m, the line y = a/b is the horizontal asymptote
• if n > m, there is no horizontal asymptote
|
| 4. Use the vertical asymptote(s) to divide the x-axis into regions. |
| |
• Graph at least one point in each region.
• Use this point to determine the position of the graph in relation to the horizontal asymptote(s).
• It may be necessary to graph additional points to get the shape of the graph.
|
| 5. Sketch the graph. |

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|