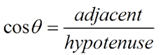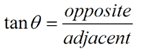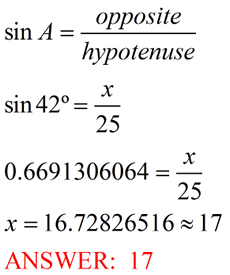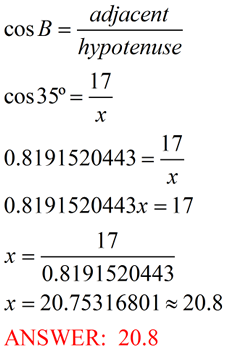|
 Remember the three trigonometric functions that we will be using. See Trigonometric Functions for more information on labeling triangles and establishing these ratios.
Remember the three trigonometric functions that we will be using. See Trigonometric Functions for more information on labeling triangles and establishing these ratios.
One of the beauties of these trigonometric functions is that you can find two missing sides of a right triangle if you are given only one side and an acute angle.

 Using Trigonometric Functions to Find a Missing Side Using Trigonometric Functions to Find a Missing Side |
How to set up and solve a trigonometry problem:
Set up the diagram:
1. Draw a diagram depicting the situation, if one is not given.
2. Place the angle degrees INSIDE the triangle.
3. Imagine standing at the reference angle.
4. LABEL the triangle with o, h, and a.
o - opposite side (the side across from you)
h - hypotenuse (across from the right angle)
a - adjacent side (the leftover side)
5. "Pair Up" the values.
The h pairs with the 25.
The o pairs with the x.
The a stands alone, which means the a is not involved in the solution to this problem.
Cross it out!
This problem deals with o and h.
|
Find x, to the nearest integer. 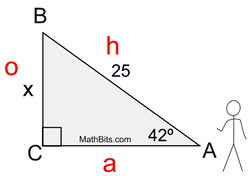
Note: Trigonometry problems usually ask for an answer to
the nearest units. |
Set up the formula:
1. Since this problem deals with o and h, we use the sine function since it also deals with o and h.
2. Replace A with the angle degrees.
3. Replace o and h with their companion terms.
4. Use your scientific/graphing calculator to determine the value of sin 42º. (On most graphing calculators, set the mode to degree and press the sin key followed by 42. Most scientific calculators will reverse this order: press 42 first, followed by the sin key.
5. Solve the equation algebraically. In this problem, cross multiply and solve for x. (When x is on top, you multiply to get the answer. When x is on the bottom, you will divide to get the answer - see example below.)
6. Round answer to desired value. |
|

In right triangle ABC, ∠C is the right angle, BC = 17 and angle B = 35º.
Find BA to the nearest tenth.
Set up the diagram and the formula in the same manner as shown above.
You should arrive at the set-up and diagram shown below. |
|
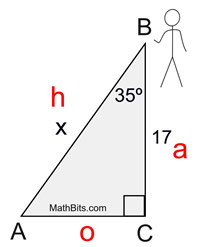 Notice that o is not "paired up" with another term, telling us that this problem deals with a and h, which is cosine.
Notice that o is not "paired up" with another term, telling us that this problem deals with a and h, which is cosine. |
Note: If you are having a problem solving the equation algebraically,
remember that when x is on the bottom, you must divide to arrive at your answer.
The division is always "divide BY the trig value decimal". |
 |
Be sure your answer MAKES SENSE!!!
The hypotenuse is always the longest side in a right triangle. So, the answer of 20.8 in the example above makes sense - as the hypotenuse it needs to be longer than the leg of 17.
|

 Trigonometric Functions on the TI-84+ Calculator Trigonometric Functions on the TI-84+ Calculator |
When first learning about trigonometric ratios, you will be working in degree mode. Since the calculator defaults to Radian mode, be sure to
set your graphing calculator to
DEGREE MODE.
Also, use the full calculator entry when working a problem. Only round when you are ready for your final rounded answer.
|
|
For help with
trig ratios on
your calculator,
click here. |
|
|

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|