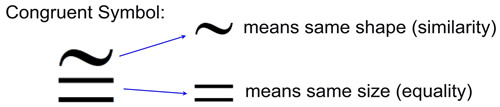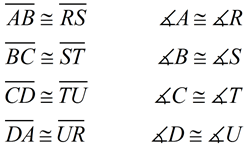def
The word "congruent" is defined in the dictionary as
"identical in form; coinciding exactly when superimposed."
The origin of the word "congruent" is from the Latin to "agree" or "to meet together".

Intuitively, congruent figures are the same size and shape.
It is possible to turn, flip and/or slide one figure so it will fit exactly on the other figure.
The areas of congruent figures are equal.
The perimeters of congruent figures are equal.
The corresponding angles of congruent figures are equal in measure.
The corresponding line segments of congruent figures are equal in length.

Various congruent figures:
Two congruent isosceles triangles. The blue triangle is a "turn" (rotation) of the red triangle.
isoscongruent
Two congruent cartoon birds. The bird on the right is a "flip" (reflection) and "slide" (translation) of the bird on the left.congruentcartoonbirds
Three congruent regular pentagons. The purple and blue pentagons are "slides" of the red pentagon.congpent
Two congruent designs. The design on the right is a "turn" (rotation) of the design on the left.congdesign
Two congruent circles. The circle on the right is a "slide" (translation) of the circle on the left.

congcircles
Two congruent shapes. The shape on the right is a "slide" (translation) of the shape on the left.congshapes

As seen in the table above, even though two figures may be duplicates of one another (congruent), they may not appear in the same positions when first viewed. After a transformation, or series of transformations, however, one figure can be moved to coincide with the other.   


Congruent geometric figures:

hint
congruent symbol

Matching Parts: When working with congruent geometric figures, locate the sides and the angles that "match up" (are in the same locations after a transformation). Sides and angles that "match up" are called corresponding sides and corresponding angles.

In congruent figures, the corresponding sides are congruent, and the corresponding angles are congruent. Notice the 8 facts known to be true about the two congruent trapezoids.
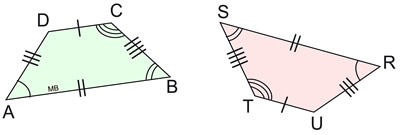
Trapezoid ABCD congruent Trapezoid RSTUcongtrap
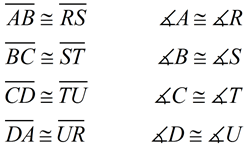trapparts
Known information regarding the corresponding parts in congruent figures is often marked on the diagrams. The corresponding congruent sides are marked with small straight line segments called hash marks. The corresponding congruent angles are marked with arcs.

The order of the letters in the names of the congruent figures displays the corresponding relationships. In this manner, even without the diagrams for our trapezoid example, you would know that ∠A would be congruent to ∠R, ∠B would be congruent to ∠S, and so on. In addition, 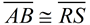ABRSbecause these segments are in the same positions in each trapezoid's name.


Thinking about "when" figures are congruent:
1. Will figures be congruent if all of the corresponding sides and all of the corresponding angles are congruent? Most definitely! Having all corresponding sides and angles congruent is the essence of figures being congruent.

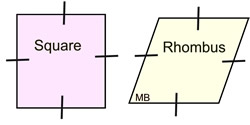
2. Will figures be congruent if all of the corresponding sides are congruent, but the corresponding angles are not congruent? The answer is "No"! Think about all of the possible situations and combinations that could occur. For example, the sides of a square and the sides of a rhombus could be congruent, but the angles of a rhombus need not be each 90ยบ.
 sqrhom

3. Will figures be congruent if all of the corresponding angles are congruent, but the corresponding sides are not congruent? The answer is "No"! Again, think about all of the possible situations. For example, the angles of a square and the angles of a rectangle are congruent, but the sides of the rectangle need not be the same length as the sides of the square.
sqrect

divider


NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".