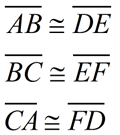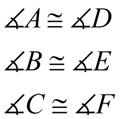|
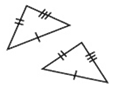
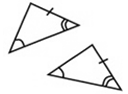
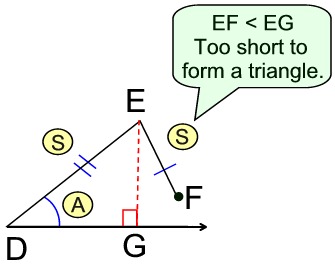
When triangles are congruent, one triangle can be moved (through one, or more, rigid motions) to coincide with the other triangle. All corresponding sides and angles will be congruent.

When triangles are congruent, six facts are always true.
|
Corresponding sides are congruent. |
Corresponding angles are congruent. |
|
|
The good news is that when proving triangles congruent, it is not necessary to prove all six facts to show congruency. There are certain ordered combinations of these facts that are sufficient to prove triangles congruent. These combinations guarantee that, given these facts, it will be possible to draw triangles which will take on only one shape (be unique), thus insuring congruency.
Methods that Prove Triangles Congruent |
The following ordered combinations of the congruent triangle facts
will be sufficient to prove triangles congruent.
If three sides of a triangle are congruent to three sides of another triangle, the triangles are congruent. |
If two sides and the included angle of one triangle are congruent to the corresponding parts of another triangle, the triangles are congruent. |
If two angles and the included side of one triangle are congruent to the corresponding parts of another triangle, the triangles are congruent.
|
AAS (or SAA)
Angle-Angle-Side |
|
|
If two angles and the non-included side of one triangle are congruent to the corresponding parts of another triangle, the triangles are congruent.
This is an extension of ASA. In ASA, since you know two sets of angles are congruent, you automatically know the third sets are also congruent since there are 180º in each triangle.
|
If the hypotenuse and leg of one right triangle are congruent to the corresponding parts of another right triangle, the right triangles are congruent. |
The "included angle" in SAS is the angle formed by the two sides of the triangle being used.
The "included side" in ASA is the side between the angles being used. It is the side where the rays of the angles overlap.
The "non-included" side in AAS can be either of the two sides that are not directly between the two angles being used. |
|
Once triangles are proven congruent, the corresponding leftover "parts" that were not used in SSS, SAS, ASA, AAS and HL, are also congruent.
CPCTC
Corresponding Parts of Congruent Triangles are Congruent.
Once the triangles are congruent, all six facts about congruent triangles will be true.
|


Methods that DO NOT Prove Triangles Congruent |
The following ordered combinations of the congruent triangle facts will NOT be sufficient to prove triangles congruent.
Let's see why these combinations DO NOT work!
|
|
This method will NOT always prove triangles congruent! |
The AAA combination will show that the triangles are the same SHAPE (similar), but will NOT show that the triangles are the same size.
Example: Consider these two equilateral triangles that satisfy the AAA combination. They are the same shape, but are not the same size. Thus, they are not congruent. (They are similar.) |
 |
Yes, it is possible that the sides "could" be the same length and the triangles would be congruent, but this would be the exception, not the rule. |
|
SSA or ASS
Side-Side-Angle |
|
This method will NOT always prove triangles congruent! |
The SSA (or ASS) combination deals with two sides and the non-included angle. This combination is humorously referred to as the "Donkey Theorem". SSA (or ASS) is NOT a universal method to prove triangles congruent since it cannot guarantee that the shapes of the triangles formed will always be the same. |
|
Let's look at the problems associated with SSA:
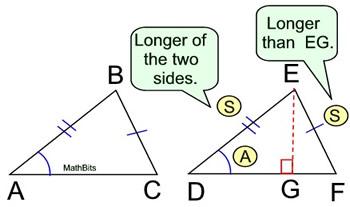
When using SSA (or ASS), the lengths of the sides being used, and their position in relation to the angle, will determine if congruent triangles will always be created. Let's see which special conditions will create congruent triangles, and which ones will not. |
 If the side which lies on one ray of the angle is shorter than the other side (not on the ray of the angle), you are safe and the two triangles will be of the same shape and size (congruent). If DE is shorter than EF, only one triangle may be drawn. If the side which lies on one ray of the angle is shorter than the other side (not on the ray of the angle), you are safe and the two triangles will be of the same shape and size (congruent). If DE is shorter than EF, only one triangle may be drawn.
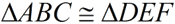 |
|
 If the side which lies on one ray of the angle is longer than the other side, and the other side is the minimum distance needed to create a triangle, the two triangles will be congruent. The minimum (shortest) distance from point E to the ray from D through F, is the perpendicular distance. Using the right angles, we can establish AAS making the triangles congruent. Or using the Pythagorean Theorem, we can find the missing side, and then use SSS, SAS, or ASA to make the triangles congruent. If the side which lies on one ray of the angle is longer than the other side, and the other side is the minimum distance needed to create a triangle, the two triangles will be congruent. The minimum (shortest) distance from point E to the ray from D through F, is the perpendicular distance. Using the right angles, we can establish AAS making the triangles congruent. Or using the Pythagorean Theorem, we can find the missing side, and then use SSS, SAS, or ASA to make the triangles congruent.
|
|
Note: This specific case of SSA is the basis for the acceptable method HL (Hypotenuse Leg) which applies only in right triangles. |
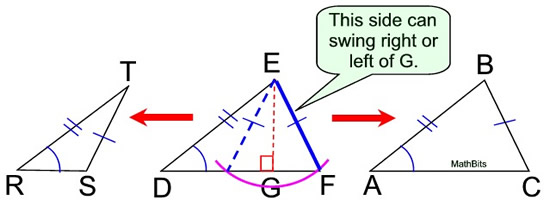
 If the side which lies on one ray of the angle is longer than the other side, and the other side is greater than the minimum distance needed to create a triangle, the two triangles will not necessarily be congruent. If the side which lies on one ray of the angle is longer than the other side, and the other side is greater than the minimum distance needed to create a triangle, the two triangles will not necessarily be congruent.
If EF is greater than EG, the diagram below shows how it is possible for  to "swing" to either side of point G, creating two non-congruent triangles using SSA. to "swing" to either side of point G, creating two non-congruent triangles using SSA. |
|
|
 If the side which lies on one ray of the angle is longer than the other side, and the other side is less than the minimum distance needed to create a triangle, no triangle can be drawn. Since no triangles are possible, no congruent triangles are possible. If the side which lies on one ray of the angle is longer than the other side, and the other side is less than the minimum distance needed to create a triangle, no triangle can be drawn. Since no triangles are possible, no congruent triangles are possible.
No Congruent Triangles |
|
Since the SSA (or ASS) method allows for the possibility of creating triangles of various shapes (or even no triangles at all), this method is not an universal method for proving triangles congruent.
|

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|