Remember -- use your compass
and straightedge only!

Square

We will be looking at TWO different constructions of a square. The first will be to construct a square given the length of one side, and the other will be to construct a square inscribed in a circle.

METHOD 1:
Given: the length of one side of the square
Construct: a square
 equiAB


STEPS:
1. Using your straightedge, draw a reference line, if one is not provided.

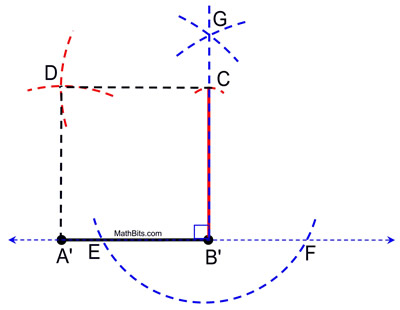
2. Copy the side of the square onto the reference line, starting at a point labeled A'.

3. Construct a perpendicular at point B' to the line through ab2.

4. Place your compass point at B', and copy the side of the square onto the perpendicular b'g. Label the end of the segment copy as point C.

5. With your compass still set at a span representing AB, place the compass point at C and swing an arc to the left.

6. Holding this same span, place the compass point at A' and swing an arc intersecting with the previous arc. Label the point of intersection as D.

7. Connect points A' to D, D to C, and C to B' to form a square.

square1

Proof of Construction: As a result of the construction of the perpendicular at B', m∠A'B'C = 90º, since perpendicular lines meet to form right angles, and a right angle contains 90º. By copying the segment length of the side of the square, ab2, we have A'B' = B'C = CD = DA'. A figure having four congruent sides and an interior angle which is a right angle, is a square.

dividerdash

METHOD 2:
Given: a piece of paper
Construct: a square inscribed in a circle.
  


STEPS:
1. Using your compass, draw a circle and label the center O.

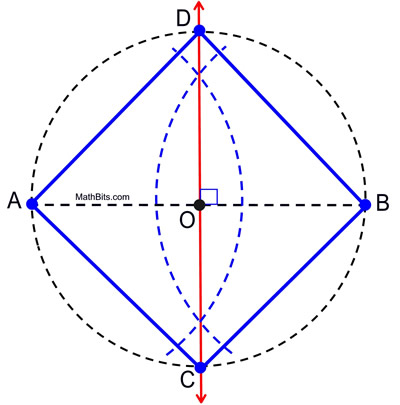
2. Using your straightedge, draw a diameter of the circle, labeling the endpoints A and B.

3. Construct the perpendicular bisector of the diameter, ab2.

4. Label the points where the bisector intersects the circle as C and D.

5. Connect points A to D to B to C to A to form the square.

square2

Proof of Construction: ab2 is a diameter of the circle since it passes through the center of the circle. From the construction of the perpendicular bisector of ab2, we know that O is the center of ab2 (and the center of the circle), making cd also a diameter of the circle. In addition, perpbisABCE. Since both ab2 and cd are diameters, we have radii AO = BO = CO = DO showing that ab2 and cd bisect each other. Since the diagonals bisect each other, ABCD is a parallelogram. And since diameters of a circle are congruent, we also know that the diagonals of ABCD are congruent and perpendicular, making ABCD a square.


divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".