Practice Page
Directions: Read carefully and choose the best answer.

1.
Write the equation of a circle whose center is at (3,-2) and has a radius of 11.
circleguy1
Choose:
 
(x - 3)2 + (y - 2)2 = 121
  (x - 3)2 + (y + 2)2 = 121
  (x - 2)2 + (y + 3)2 = 121
  (x + 2)2 + (y - 3)2 = 121



2.
Given the equation of a circle,
(x - 5)2 + (y + 3)2 = 196,
state the coordinates of the center and the radius.
circleguy2
Choose:
 
center (-5,3), radius 13
 
center (5,-3), radius 13
  center (-5,3), radius 14
  center (5,-3), radius 14



3.
State the coordinates of the center and the radius of a circle whose equation is
x2 + y2 + 2x - 4y - 11 = 0 .
circleguy3
Choose:
 
center (-1,2), radius 4
 
center (-1,2), radius rad11
  center (1,-2), radius rad11
  center (1,-2), radius 4



4.
Graph the circle: x2 + 10x + y2 - 6y = - 30.



circleguy4



5.
Write the equation of a circle whose center is (-4,8) and passes through the point (-2,-1).
circleguy5
Choose:
 
(x - 4)2 + (y - 8)2 = 40
 
(x + 4)2 + (y - 8)2 = 85
  (x + 2)2 + (y + 1)2 = 40
  (x + 2)2 + (y + 1)2 = 85



6.
Write the general equation of a circle that is tangent to the x-axis, with a center located at (4,-6).

circleguy6



7.
Show that the point 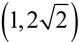2rad2 lies on a circle whose center is the origin and contains the point (0,3).


circleguy7



8.
The equation x2 + y2 - 12x - 8y + 27 = 0 is equivalent to:
circleguy8
Choose:
 
(x - 6)2 + (y - 4)2 = 25
 
(x + 6)2 + (y + 4)2 = 25
  (x - 6)2 + (y - 4)2 = 27
  (x + 6)2 + (y + 4)2 = 3rad3



9.
A regular hexagon ABCDEF with a side length of 4 units is centered at G(5,3). State the general equation of the circle circumscribing the hexagon.
circlehexagon
Choose:
 
x2 + y2 - 10x - 6y + 30 = 0
 
x2 + y2 + 10x + 6y + 30 = 0
 
x2 + y2 - 10x - 6y + 18 = 0
 
x2 + y2 + 10x + 6y + 18 = 0



10.
Which of the equation choices could represent the circle shown on the graph?
(Check all that apply, and hit SUBMIT!)
 

(x - 5)2 + (y - 6)2 = 9

x2 + y2 = 9
x2 + y2 - 10x - 12y + 52 = 0

(x + 5)2 + (y - 6)2 = 9

x2 + y2 - 10x - 12y - 52 = 0

 x2 + y2 = 10x + 12y

x2 + y2 - 10x - 12y = -52
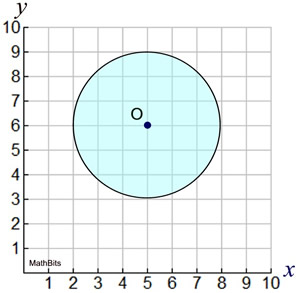circlechoice
(Assume a radius of integer length.)

 

 
11.
The equation x2 + y2 - 6x + 4y = d
describes a circle.
a) Determine the y-coordinate of the center of the circle.
b) The radius of the circle is 6 units. What is the value of "d" in the given equation.

circleguy9



12.
Given circle: x2 - 9x + y2 = 4.75
In center-radius form, this equation will be written as:
circleguy10
Choose:
 
(x + 3)2 + y2 = 25
 
(x - 4.5)2 + y2 = 25
 
(x - 3)2 + y2 = 20.25
 
(x +4.5)2+ y2 = 22.5625

divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".