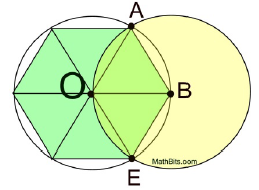
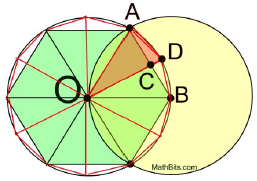
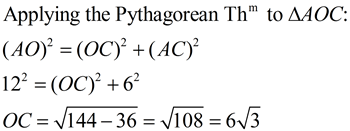GeometrySmallLogo

Circumference of Circle

 


 The perimeter of a circle is called the circumference and is the linear distance around the edge of a circle. The circumference of a circle is proportional to its diameter, d, and its radius, r, and relates to the famous mathematical constant, pi (π).
C = π • d = 2π • r
Circumference is from Latin meaning "carrying around".

If you "unroll" the outer edge of a circle, it will form a straight segment whose length is three diameters plus a little bit more. You will get this same result using a circle with extremely small radius length as you will using a circle with enormously large radius length. It was the need to understand this constant length that led to the constant pi (π = 3.14159...).

unrolling


Derivation of the Circumference Formula:
As we have seen, the formula for the circumference of a circle is related to the value of π, and is expressed as C = π • d = 2π • r. But, how did the values of C and π come to be related to one another in this manner? Using an approach developed by Archimedes, we can show the relationship.
circ1
Draw circle O. From point B, on the circle, draw another circle with center at B, and radius OB. The intersections of the two circles at A and E form equilateral triangles AOB and EOB, since they are composed of 3 congruent radii. Extend the radii forming these triangles through circle O to form the inscribed regular hexagon with 6 equilateral triangles.
The perimeter of the hexagon is 6 times the radius of the circle. But it can be seen that the actual circumference of the circle is "bigger" than this perimeter. Thus, the circumference of the circle must be more than 6r. [Think: In C = 2π • r, our findings show that π must be slightly bigger than 3, which we know to be true. 2π r > 6r ⇒ 2π r > 2•3r ⇒ π > 3]
circ2
Attempting to get closer to the actual value of π, we bisect each of the central angles of the hexagon to obtain the sides of a 12-sided figure (a dodecagon). Notice that we are getting closer to the actual circumference of the circle.
Notice that OC is the length of the altitude of the equilateral triangle, making ∠OCA a right angle, and forming ΔAOC congruent to ΔBOC.

For ease of computation, let's say that the length of the radius, AO, in this diagram equals 12 units. We know that AB = OA (radius), so AC, which is half of AB, equals 6.

circ3
 circ4

The length AD (the side of the dodecagon) can be determined by Pythagorean Thm in ΔACD.

circ5
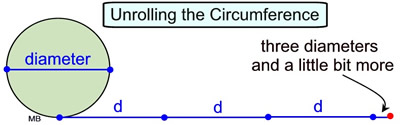
The perimeter of dodecagon = 12•(6.211) = 74.532. The circumference of circle O must be greater than 74.532, or greater than 6.211r. This shows π must be a little greater than 3.105.
We are getting closer to the actual value of π !

If we continue to increase the number of sides of the inscribed polygon, we will get closer to the actual circumference of the circle, and our solution for π will get closer to the actual value of 3.14159...

divider

 

Area of Circle

 

 

Like circumference, the area of a circle also deals with pi (π).
A = π • r2

Derivation of the Area Formula:
You have seen the derivation of this formula in past years. Here is a refresher:

We start knowing that the circumference of a circle is C = 2π • r, and that the area of a rectangle is A = bh.

A circle is divided into congruent sectors (pie slices). The sectors are pulled out of the circle and are arranged as shown in the middle diagram. The length across the top (over the curved arcs) is half of the circumference. When placed in these positions, the sectors form a parallelogram. The larger the number of sectors that are cut, the less curvy the arcs will appear and the more the shape will resemble a parallelogram. As seen in the last diagram, the parallelogram ca be changed into a rectangle by slicing half of the last sector and placing it to the far left.

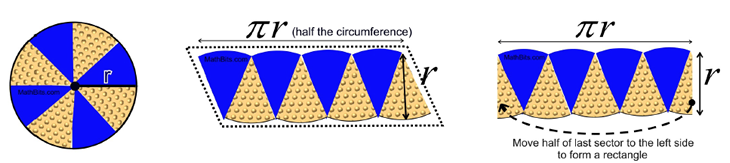
areapi

The area of the rectangle is A = bh.
Thus, the area of the sectors that make up the rectangle is πr • r = π • r2.


divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation and is not considered "fair use" for educators. Please read the "Terms of Use".