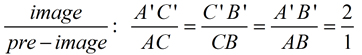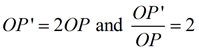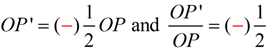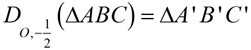|
For an intuitive review of dilations, see the Refresher section Transformations: Dilations.
Now, let's expand that knowledge of dilations in relation to high school geometry.
The unit Rigid Transformations examined transformations where the pre-image and the image are congruent (the same size and the same shape). These rigid transformations include reflections, translations and rotations, but NOT dilations.
A dilation is a transformation, but NOT a "rigid" transformation (or isometry) because the image is NOT the same size as the pre-image. Remember that rigid transformations preserve distance (length), but dilations do not. Under a dilation, a pre-image and its image are similar, but not congruent.
In plain English, a dilation is a transformation that produces an image that is the same shape as the original, but is a different size.
|
(It is possible, but unusual, to have a dilation scale factor of 1 creating congruent figures.)
Our discussion of dilations will focus on the scale factor NOT being the number 1.
• The description of a dilation includes the scale factor (or ratio) and the center of the dilation.
•
The center of a dilation is a fixed point in the plane about which all points are expanded or contracted. The center is the only invariant (not changing) point under a dilation, and can be located inside, outside, or on a figure. Dilations never move the center point of the dilation.

Let's take a look an example of dilations in relation to triangles.
|
 |
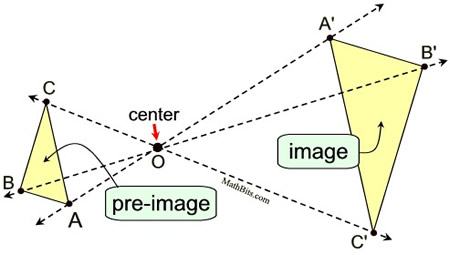
Point O is the center of this dilation.
In this dilation, of scale factor 2 mapping ΔABC to ΔA'B'C', the distances from O to the vertices of ΔA'B'C' are twice the distances from O to ΔABC.
After a dilation, the pre-image and image have the same shape but not the same size.
|
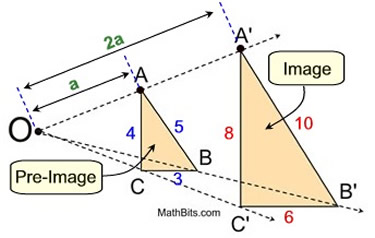
In a dilation, the sides of the pre-image and the corresponding sides of the image are proportional.
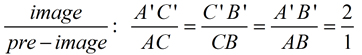 The sides of these triangles are ΔABC (sides 3,4,5) and ΔA'B'C' (sides 6,8,10).
The sides of these triangles are ΔABC (sides 3,4,5) and ΔA'B'C' (sides 6,8,10).
 |
The "notation form" for this diagram, will be:
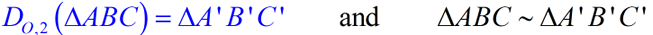
This notation reads, "the dilation, centered at O with a scale factor of 2, of ΔABC will create ΔA'B'C'."
This dilation is an "enlargement" as the triangle created is "bigger" than the starting triangle.
Also, this dilation makes ΔABC and ΔA'B'C' similar.

In this example, ΔD'E'F' is the image of ΔDEF (dilation center O, scale factor ½).
Distances from the center:
 |
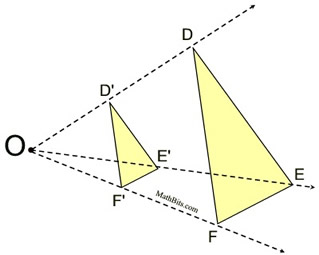 |
This dilation is a "reduction" as the triangle created is "smaller" than the starting triangle.

Formal Definition
 |
A dilation is a transformation, DO,k , with center O and a scale factor of k that maps O to itself, and any other point P to P'. |
The center O is a fixed point. P' is the image of P.
Points O, P and P' are collinear (on the same line).
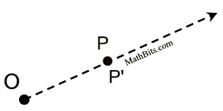
Scale factor k: OP' = k•OP or 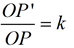 |
|

Properties of Dilations
Properties preserved under a dilation from the pre-image to the image.
1. angle measures (remain the same)
2. parallelism (parallel lines remain parallel)
3. collinearity (points remain on the same lines)
4. orientation (lettering order remains the same)
----------------------------------------------------------
5. distance is NOT preserved (lengths of segments are NOT the same in all cases, except a scale factor of 1).
A dilation is NOT a rigid transformation (isometry). |
|
Scale Factor, k:
• If k > 1, enlargement.
• If 0 < k < 1, reduction.
• If k = 1, congruent.
Note: If k < 0, the image will be placed on the opposite side of the center and rotated 180º.
Dilations create similar figures!
|

Scale Factors (k):
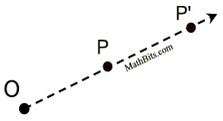
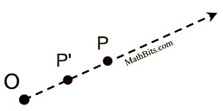
The examples below show what will happen to the dilation of point P,
from a dilation center at O, as the scale factor changes. Notice the location of P'.
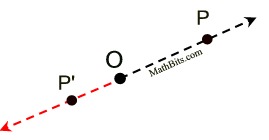
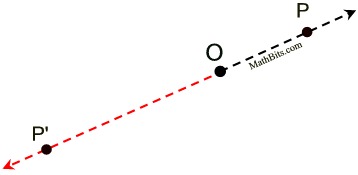
What happens when scale factor k is a negative value?
If the value of scale factor k is negative, the dilation takes place in the opposite direction from the center of dilation on the straight line containing the center and the pre-image point. (This "opposite" placement may be referred to as being a " directed segment" since it has the property of being located in a specific "direction" in relation to the center of dilation.)
Let's see how a negative dilation affects a triangle:
Notice that the "image" triangles are on the opposite side of the center of the dilation (vertices are on opposite side of line from O). Also notice that the triangles have been rotated 180º.
k = -½
Directed Segments - Reduction
Center of dilation O,
scale factor of -½

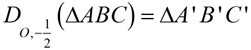
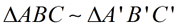
|
|
k = -2
Directed Segments
Enlargement
Center of dilation O
scale factor of -2
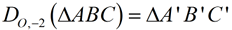
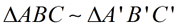
This dilation cannot be expressed as simply a rotation of 180º centered at O, because the triangle changed size.
|
|
|
Did you notice the connection between negative dilations and rotations?
When the scale factor was k = -1, the triangles were congruent and the negative scale factor produced the same result as a rotation of 180º centered at O.
BUT ...
We cannot describe dilations such as k = -½ or k = -2 as simply a "rotation of 180º about O", because these transformations did not preserve length (size), as rotations must do.
Remember, rotations are "rigid" transformations (no changes in size).
We can, however, express these dilations as a composition of transformations involving a rotation. For example, when k = -½, we can also describe that transformation as a dilation of scale factor +½ combined with a rotation of 180º (both centered at O).

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|