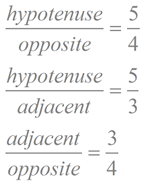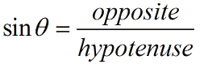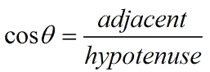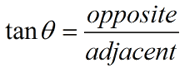A literal translation of "trigonometry" is "triangle measure".

A trigonometric function relates one acute angle (non-right angle) of a right triangle to the ratio of the lengths of any two sides of the triangle.

As we saw with the 30º-60º90º and 45º-45º-90º triangles, once one angle in a right triangle is known, all right triangles with that same acute angle will be similar by Angle-Angle (AA). Such consistency allows for the creation of ratios regarding the lengths of the sides of such triangles.

Labeling:

 

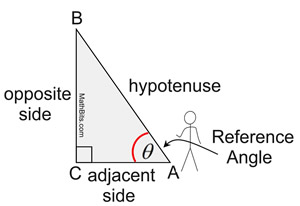
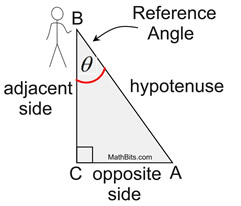
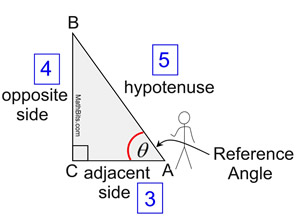
Trigonometric functions can operate with either of the two acute angles of a right triangle. A certain labeling is used to refer to the components of these triangles. This labeling is based upon which of the two acute angles is being used in the problem. Before you can use a trigonometric function, you need to have a "point of reference" from which to work, which is called your Reference Angle.

Think of yourself as standing at the Reference Angle (the angle used in your problem). You know where the hypotenuse is located. The leg across from you is called the "opposite side", and the left-over leg is called the "adjacent side" (the leg "next" to you).

bullet Notice how the labeling changes when the Reference Angle location changes.
label1
label2
It is customary (but not mandatory) to use the Greek letter "theta" (θ)
as a label for a reference angle.

dividerdash

How many ratios?

 

So, how many ratios pertaining to the sides of the triangle are possible? Let's take a look:
label3 What possible ratios
of the sides exist?
label4		 If we flip these three ratios over, we have three more:
label5
These will be studied in a later course.

dividerdash


Naming the ratios:

 

The ratios that we established above have specific "names". The first three ratios we created are called sine, cosine and tangent and are referred to as trigonometric functions.

Sine (sin)
Cosine (cos)
Tangent (tan)
sine
cosein
tangent

We will be abbreviating the statements above to state:
name1
There are memory tricks to help you remember these statements:
"sohcahtoa" (simply remember the order as read from left to right)
"oscar had a heap of apples" (remembering sin, cos, tan in order)
"oh heck, another hour of algebra!"

beware Trigonometric functions work ONLY in right triangles!

See how to solve for a missing side or a missing angle using trigonometry.


divider


NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".