The sieve of Eratosthenes (276 BC - 194 BC) is an ancient method for finding all prime numbers up to a specific given value. The concept is to progress through a chart of consecutive integers removing the multiples of each prime number, starting with the first prime number 2.

How it Works:
Start with a chart of consecutive positive integers. Multiples of the prime numbers will be crossed out throughout the chart. This chart will be from 1 to 100.

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100

1.
 Cross out 1 (it is not a prime).
2.
 Start with 2. Circle 2 as a prime number, but cross out all multiples of 2 (every even number).
3.
 Now, move to the next prime 3. Circle 3 as a prime number, but cross out any multiple of 3 remaining in the chart.
4.
 Now, move to 5. Circle 5 as a prime number, but cross out any multiple of 5 remaining in the chart.
5.
 Now, move to 7. Circle 7 as a prime number, but cross out any multiple of 7 remaining in the chart.
6.
 Continue this process for each prime number you encounter on the chart. Remember that a prime is divisible only by 1 and itself.
7.
 The circled values in the chart are the prime numbers between 1 and 100.

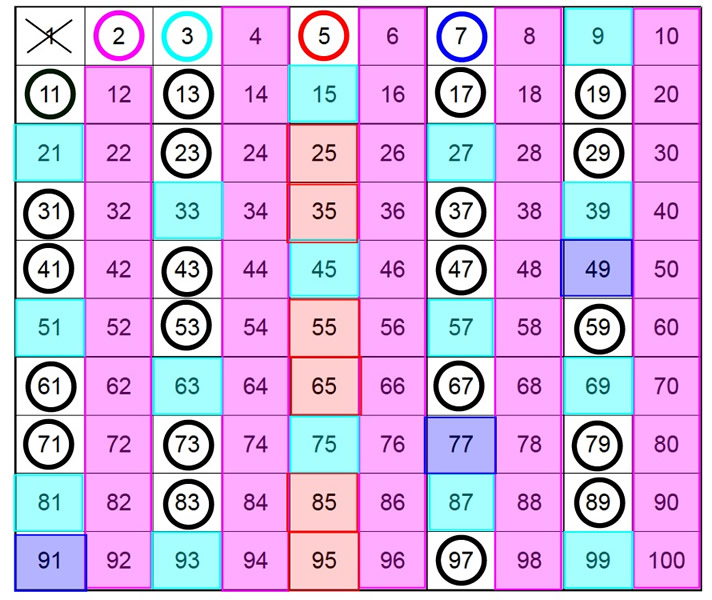
sievepic

The multiples of the values circled in black had already been colored,
or do not exist in this small chart.
The prime numbers between 1 and 100 are:
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97

divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".