|
This is a partial listing of the more popular rules (theorems, postulates, and properties) that you will be using in your study of Geometry.
First a few words that refer to types of geometric "rules":
• A theorem is a statement (rule) that has been proven true using facts, operations and other rules that are known to be true. These are usually the "big" rules of geometry. A short theorem referring to a "lesser" rule is called a lemma.
• A corollary is a follow-up to an existing proven theorem. Corollaries are off-shoots of a theorem that require little or no further proof.
• A postulate (or axiom) is a statement (rule) that is taken to be true without proof. Euclid derived many of the rules for geometry starting with a series of definitions and only five postulates.
• A property is a quality or characteristic belonging to something.
For example, the real numbers have the associative, commutative and distributive properties.
|
Your textbook (and your teacher) may want you to remember these "rules" with slightly different wording.
Be sure to follow the directions from your teacher. |
 Angles: Angles:
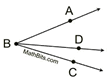
Adjacent Angles |
Two angles that share a common vertex, a common side, and no common interior points (don't overlap).
m∠ABD and m∠DBC are adjacent. m∠ABC and m∠DBC are not adjacent |
 |
|
Linear Pair |
Two adjacent angles whose non-common sides for a straight line. |
Straight Angles |
All straight angles are congruent (equal in measure).
(They all have a measure of 180º.)
|
Vertical Angles |
Vertical angles are congruent (equal in measure).
m∠1 = m∠2
m∠3 = m∠4 |
|
|
Triangle Interior Sum |
The sum of the measures of the interior angles of a triangle is 180º.
|
Exterior Angle |
The measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles.
|
Angles forming a straight line |
|
Angles around a point |
|
Complementary Angles |
Two angles the sum of whose measures is 90º. |
Supplementary Angles |
Two angles the sum of whose measures is 180º. |
 Triangles: Triangles:
Pythagorean Theorem |
c2 = a2 + b2
In a right triangle, the square of the hypotenuse equals the sum of the square of the lengths of the legs. |
Sum of Two Sides |
The sum of the lengths of any two sides of a triangle must be greater than the third side. |
Longest Side |
In a triangle, the longest side is across from the largest angle. |
Largest Angle |
In a triangle, the largest angle is across from the longest side |
Congruent Triangles |
Triangles that are congruent if there corresponding angles are congruent and their corresponding sides are congruent. |
Short-cuts to verify congruent triangles |
SSS, ASA, AAS, SAS, HL(in right triangles) |
Angle-Angle (AA) Similarity |
If two angles of one triangle are congruent to two angles of another triangle, the triangles are similar. |
Sides of Similar Δs |
Corresponding sides of similar triangles are in proportion. |
 Parallels: Parallels:
Corresponding Angles |
If two parallel lines are cut by a transversal, then the pairs of corresponding angles are congruent. |
Alternate Interior Angles
|
If two parallel lines are cut by a transversal, then the alternate interior angles are congruent. |
Alternate Exterior Angles |
If two parallel lines are cut by a transversal, then the alternate exterior angles are congruent. |
Interiors on Same Side |
If two parallel lines are cut by a transversal, the interior angles on the same side of the transversal are supplementary. |
|
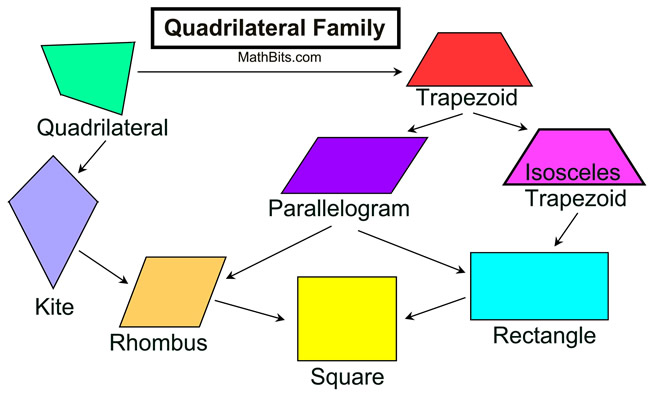
 Quadrilaterals: Quadrilaterals:
Quadrilateral |
• is a four sided polygon
•
a figure with exactly four sides
|
Parallelograms |
• is a quadrilateral w/ both pairs of opposite sides parallel
• opposite
sides are equal in length |
Rectangle |
• is a parallelogram with 4 right angles
• two pairs of parallel sides
• opposite sides of equal length |
Rhombus |
• is a parallelogram with all 4 sides of equal length
• two pairs of parallel sides
|
Square |
• is a parallelogram with 4 sides of equal length and 4 right angles
• two pairs of parallel sides |
Trapezoid |
• quadrilateral with at least one pair of parallel sides
|
Isosceles Trapezoid |
• is a trapezoid with congruent base angles
• at least one pair of parallel sides
• legs congruent
|
Kite |
• is a quadrilateral with two sets of adjacent sides equal
• not a trapezoid and not a parallelogram
|
 |
 Area (A), Volume (V), Surface Area (SA): Area (A), Volume (V), Surface Area (SA):
Rectangle |
Arectangle = l × w = b • h
l= length; w = width; b = base; h = height |
Parallelogram |
Aparallelogram = b • h |
Triangle |
AΔ = ½ • b• h |
Trapezoid |
Atrapezoid = ½ h (b1 + b2) or decompose |
Regular Polygon |
Aregular polygon = ½ • a • p
a = apothem; p = perimeter |
Circle (circumference) |
C = 2πr = πd
r = radius; d = diameter
|
Circle (area) |
Acircle = πr2 |
Rectangular Solid
(also called right rectangular prism) |
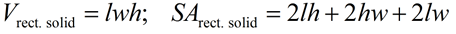 SA
SA formula assumes a "closed box" with all 6 sides. |
Cube
[special case of rectangular solid with all edges equal) |
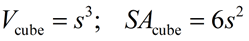 SA
SA formula assumes a "closed box" with all 6 sides. s = side |
Cylinder |
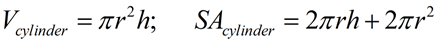 SA
SA formula assumes a "closed container" with a top and a bottom. |
Cone |
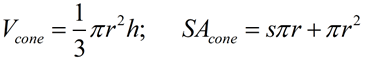 SA
SA formula assumes a "closed container", with a bottom. s = slant height |
Sphere |
|
Right Prism
(rectangular or triangular) |
Vright prism = B • h; SA = 2B + p • h
B = area of the base; h = height; p = perimeter of base |
Pyramid
[assuming all of the faces (not the base) are the same] |

B = area of the base; h = height; p = perimeter of base; s = slant height
|
|

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|