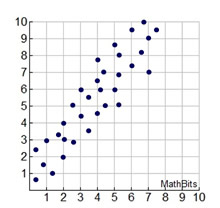
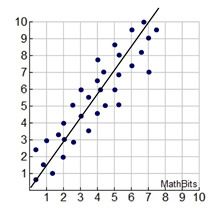
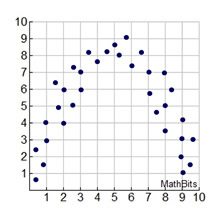
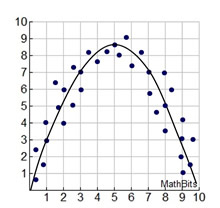
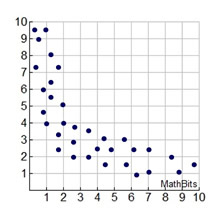
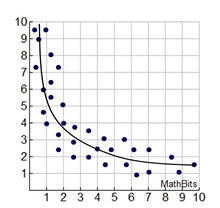
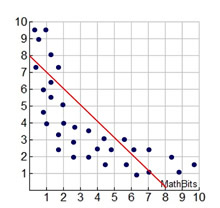
We have seen how data can often be represented by a stratight line (linear association).
Sometimes, however, a "straight line" is not the best shape to represent the data.
There are actually, different types of "curves" that can be used
to model data, referred to as non-linear associations.

Non-linear Scatter Plots
Plots that DO NOT resemble a straight line.
For this level course, you should be able to describe
a pattern as non-linear by looking at the scatter plot.

 stump
Linear:

dataline1

This scatter plot forms a straight line. The points are rising from left to right across the graph in a linear manner.

dataline2

A line can be drawn with approximately the same number of dots above and below the line.
Obviously non-Linear:

dataparabola2

This scatter plot clearly does not form a straight line. First the dots go up, but then they turn and go back down.

dataparabola1

While a "line" of best fit is not possible with this scatter plot, it may be possible to draw a "curve" of best fit.
Not Obviously non-Linear:

dataexp2

It is not easy to determine whether this scatter plot is linear or not, but it is non-linear. See *Hint below.

dataexp1

A curve can be fitted to this data with approximately the same number of dots above and below the curve.

* Hint:
Let's examine that last scatter plot (from above) again.
What if we think it is linear?

If you draw a line on a curved scatter plot and the dots collect in mass only on one side of the line for a portion of the graph, the scatter plot is mostly likely non-linear.
datalineblack

Notice how a group of the dots are only to the left (or below) the line for a section of the graph.. CLUE: This is non-linear.
datalinered

Even if we move the line to a new
position, we still have a mass of the
dots on one side of the line only.

beware It may not always be obvious from looking at a scatter plot which shape (curve) will be the best fit. For a "curve of best fit" we still try to keep the same number (or approximately the same number) of dots above the curve as below the curve, as we did with a "line of best fit".

Some situations may require more investigation before deciding upon a possible shape (curve), and some situations may not be modeled by any specific shapes (curves). Finding a "line of best fit" by hand is possible, but finding a "curve of best fit" is much more difficult. So, it upcoming courses, we will call for help from our graphing calculator to assist with "curves of best fit".


divider


NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".

Topical Outline | JrMath Outline | MathBitsNotebook.com | MathBits' Teacher Resources
Terms of Use    Contact Person: Donna Roberts

Copyright © 2012-2025 MathBitsNotebook.com. All Rights Reserved.