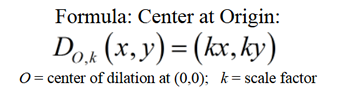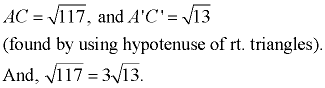|
The dictionary lists the definition of the word dilate to mean
"make or become wider, larger, or more open".
In mathematics, a dilation changes the size of a figure.
The figure may become larger or smaller, but the shape of the figure will not change.
|
The word "dilate" is often heard in relation to the human eye.
"The pupils of the eyes were dilated."
As light hits the eye, the pupil enlarges or contracts depending upon the amount of light.
|
Dilations can be seen, in a variety of situations:
Photography |
Arts and Crafts |
Graphing Calculator |
School or holiday picture packages offer the same photograph in a variety of sizes, from large to medium to small wallet size photos.
 |
Russian nesting dolls are a set of wooden dolls of decreasing size placed inside one another. After the smallest doll, each doll is an enlargement of its inside doll.
 |
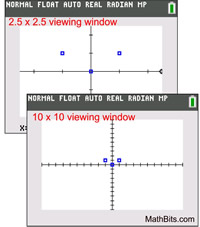
The zoom feature will enlarge or reduce the viewing window.
 |
Food Service |
Logos |
Grow Toys |
Soft drink containers come in a variety of sizes. While some are of different shapes, others are simply enlargements. |
Product logos can come in a variety of sizes, such as these pizza shop logos on their small, medium and large boxes. |
Grow toys are sponge/foam toys that "grow" when placed in water. Some grow quickly, while others grow over several days. 
|
Dilations are enlargements
(or reductions)! |
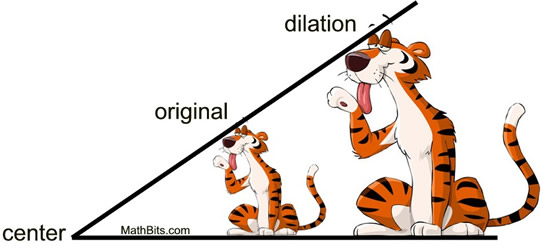
The description of a dilation includes the scale factor
and the center of the dilation.
The scale factor may also be referred to as the scalar factor.
If the larger tiger is twice as tall as the smaller tiger, the scale factor is 2.
If the scale factor is greater than 1, the image is an enlargement.
If the scale factor is between 0 and 1, the image is a reduction.
In the diagram below, A'B'C'D' is the image of ABCD under a dilation.
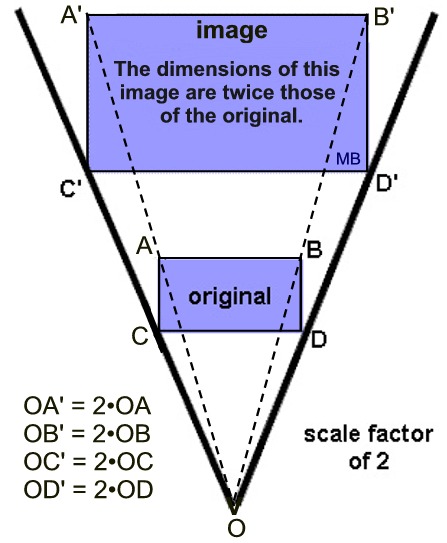
A figure and its dilation are similar figures.
Rectangle ABCD (shown above) is similar to rectangle A'B'C'D'.
The distance from the center of the dilation to each vertex point of the image is equal to the distance from the center of the dilation to each corresponding vertex point of the original figure times the scale factor. The length of each side of the image is equal to the length of the corresponding side of the original figure multiplied by the scale factor.

A dilation is a transformation that produces an image that is the same shape as the original, but is a different size. The description of a dilation includes the scale factor (constant of dilation) and the center of the dilation. The center of a dilation is a fixed point in the plane about which all points are expanded or contracted. The center is the only invariant (not changing) point under a dilation, and may be located inside, outside, or on a figure.
• A dilation that creates a larger image is called an enlargement (or expansion).
• A dilation that creates a smaller image is called a reduction (or contraction).
• A dilation is NOT a rigid transformation. (Rigid transformations preserve length, and dilations do not.)
|
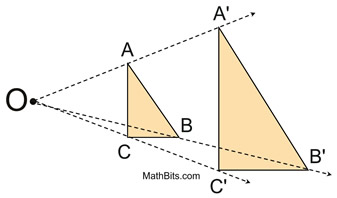 |
• A description of a dilation includes the scale factor (or ratio) and the center of the dilation.
• The center of dilation is a fixed point in the plane.
•
If the scale factor is greater than 1, the image is an enlargement. It expands.
• If the scale factor is between 0 and 1, the image is a reduction. It contracts.
• If the scale factor is 1, the figure and the image are the exact same size (congruent).


Dilations in the coordinate plane:
Most dilations in the coordinate plane use the origin, (0,0), as the center of the dilation.
The center of the dilation will be indicated within the problem (or within the notation).
 Dilation scale factor 2:
 |
Starting with ΔABC, draw the dilation image of the triangle with a center at the origin and a scale factor of two.
Notice that every coordinate of the original triangle has been multiplied by the scale factor (x2).
Dilations involve multiplication!
Dilation with scale factor 2, multiply by 2.
Center at the origin.
(x, y) → (2x,2y) |
|
 Dilation scale factor ½:
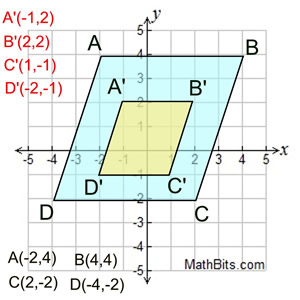 |
Starting with quadrilateral ABCD (blue), draw the dilation image of the quadrilateral with a center at the origin and a scale factor of ½.
Notice that every coordinate of the original ABCD has been multiplied by ½.
Dilation with scale factor ½, multiply by ½.
Center at the origin.
(x, y) → (½x, ½y ) |
REMINDER: Multiplying by ½ is the same as dividing by 2. |
In this problem, the center of the dilation is NOT at the origin.
 Dilation not at origin:
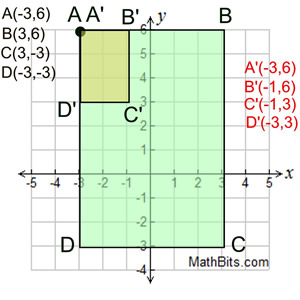 |
Starting with rectangle ABCD (green), draw the dilation image of the rectangle with the center of dilation at point A and a scale factor of 1/3
Notice that point A and its image are the same.
You must observe the distances (lengths) from the center of the dilation at point A to the other points B, C and D. The dilation image will be 1/3 of each of these distances (or lengths).
AB = 6, so A'B' = 2.
AD = 9, so A'D' = 3. Now, draw the image rectangle.
FYI:
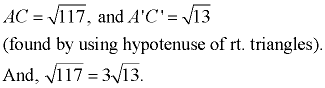
So, the diagonal of the image is also 1/3 of the original diagonal.
For a dilation not at the origin,
measure the distances. |
|


NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|